Principle of differential interference
Differential interference is a method based on the principle of optical interference to visualize small topographic features with high contrast.
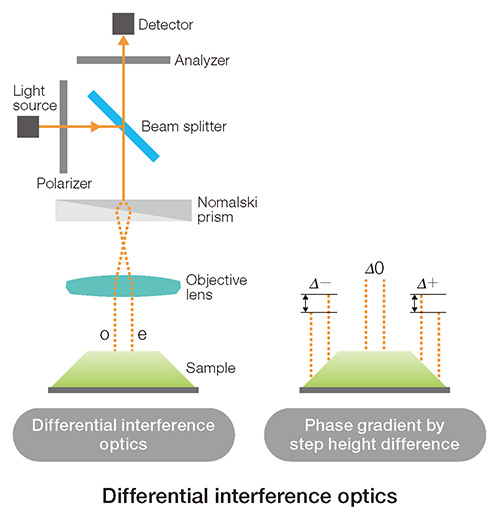
Differential interference optics
Shown below is a schematic of optical path for a reflection-type differential interference optical system. It uses a standard epi-illumination microscope in combination with a polarizer, an analyzer, and a Nomarski prism. The ray of monochromatic light from the light source gets transformed by a polarizer into a linearly polarizated beam and passes through a Nomarski prism where it is changed into two mutually orthogonal linearly polarized rays (ordinary ray: 0, extraordinary ray: e). A small gap is created between the two optical paths in this process. The rays hit two separate points on the sample, and the reflected rays pass the Nomarski prism again by travelling the same optical paths. The ordinary ray and the extraordinary ray pass the analyzer and, finally, their polarized components cause interference and reach the photodetector.
Mechanism of highlighting small height difference with brightness contrast
If there is a height difference between the two points of incidence for ordinary and extraordinary rays, it leads to a phase difference between the rays and changes the intensity of interference caused by them. If the sample has a height gap on the surface, as shown in the example below, it enhances the image's brightness contrast and highlights small topographic features. You can visualize extremely small height differences that cannot be seen with an ordinary optical system by enhancing the image's brightness contrast in the Z direction with differential interference.
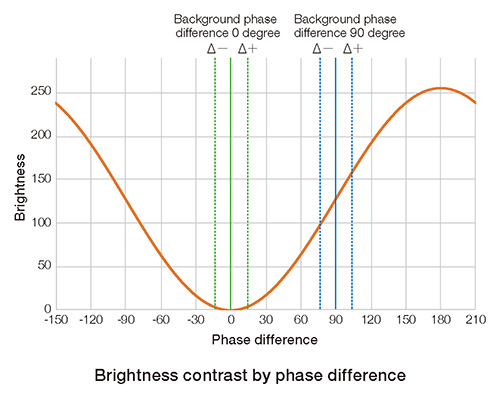
Nomarski prism and background phase
The brightness contrast provided by differential interference depends not only on the phase difference originating from the height gap in the sample but also on the total phase difference including the background phase enhanced by the Nomarski prism. You can make an optimal setting of background phase for your sample by adjusting the Nomarski prism position.
For example, when you set the background phase at 90 degrees as shown in the picture below, the brightness contrast is enhanced to make the image brighter with Δ+ and darker with Δ-. You can use Δ+ and Δ- differently in this case. When the background phase is at 0 degree, both Δ+ and Δ- cause the brightness contrast to make the image brighter. In this case, it is not possible to distinguish convex from concave, but the brightness contrast ratio increases. If you want to make a convex/concave judgment, set the background phase at 90 degrees. If you want to visualize small height differences, set it at 0 degree. In this manner, you can optimize the Nomarski prism setting. If you use white light, you can see the interference in color because a different wavelength of light creates a different degree of phase difference. You can change the background color by changing the background phase.
You might also be interested in





